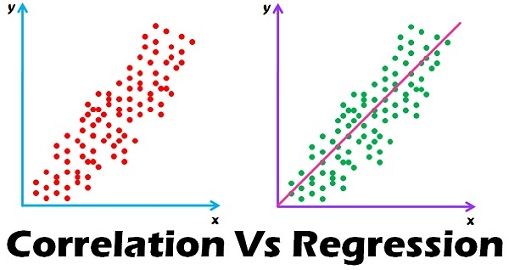
Correlation and Regression are the two analysis based on multivariate distribution. A multivariate distribution is described as a distribution of multiple variables. Correlation is described as the analysis which lets us know the association or the absence of the relationship between two variables ‘x’ and ‘y’. On the other end, Regression analysis, predicts the value of the dependent variable based on the known value of the independent variable, assuming that average mathematical relationship between two or more variables.
The difference between correlation and regression is one of the commonly asked questions in interviews. Moreover, many people suffer ambiguity in understanding these two. So, take a full read of this article to have a clear understanding on these two.
Content: Correlation Vs Regression
Comparison Chart
| Basis for Comparison | Correlation | Regression |
|---|---|---|
| Meaning | Correlation is a statistical measure which determines co-relationship or association of two variables. | Regression describes how an independent variable is numerically related to the dependent variable. |
| Usage | To represent linear relationship between two variables. | To fit a best line and estimate one variable on the basis of another variable. |
| Dependent and Independent variables | No difference | Both variables are different. |
| Indicates | Correlation coefficient indicates the extent to which two variables move together. | Regression indicates the impact of a unit change in the known variable (x) on the estimated variable (y). |
| Objective | To find a numerical value expressing the relationship between variables. | To estimate values of random variable on the basis of the values of fixed variable. |
Definition of Correlation
The term correlation is a combination of two words ‘Co’ (together) and relation (connection) between two quantities. Correlation is when, at the time of study of two variables, it is observed that a unit change in one variable is retaliated by an equivalent change in another variable, i.e. direct or indirect. Or else the variables are said to be uncorrelated when the movement in one variable does not amount to any movement in another variable in a specific direction. It is a statistical technique that represents the strength of the connection between pairs of variables.
Correlation can be positive or negative. When the two variables move in the same direction, i.e. an increase in one variable will result in the corresponding increase in another variable and vice versa, then the variables are considered to be positively correlated. For instance: profit and investment.
On the contrary, when the two variables move in different directions, in such a way that an increase in one variable will result in a decrease in another variable and vice versa, This situation is known as negative correlation. For instance: Price and demand of a product.
The measures of correlation are given as under:
- Karl Pearson’s Product-moment correlation coefficient
- Spearman’s rank correlation coefficient
- Scatter diagram
- Coefficient of concurrent deviations
Definition of Regression
A statistical technique for estimating the change in the metric dependent variable due to the change in one or more independent variables, based on the average mathematical relationship between two or more variables is known as regression. It plays a significant role in many human activities, as it is a powerful and flexible tool which used to forecast the past, present or future events on the basis of past or present events. For instance: On the basis of past records, a business’s future profit can be estimated.
In a simple linear regression, there are two variables x and y, wherein y depends on x or say influenced by x. Here y is called as dependent, or criterion variable and x is independent or predictor variable. The regression line of y on x is expressed as under:
y = a + bx
where, a = constant,
b = regression coefficient,
In this equation, a and b are the two regression parameter.
Key Differences Between Correlation and Regression
The points given below, explains the difference between correlation and regression in detail:
- A statistical measure which determines the co-relationship or association of two quantities is known as Correlation. Regression describes how an independent variable is numerically related to the dependent variable.
- Correlation is used to represent the linear relationship between two variables. On the contrary, regression is used to fit the best line and estimate one variable on the basis of another variable.
- In correlation, there is no difference between dependent and independent variables i.e. correlation between x and y is similar to y and x. Conversely, the regression of y on x is different from x on y.
- Correlation indicates the strength of association between variables. As opposed to, regression reflects the impact of the unit change in the independent variable on the dependent variable.
- Correlation aims at finding a numerical value that expresses the relationship between variables. Unlike regression whose goal is to predict values of the random variable on the basis of the values of fixed variable.
Video: Correlation Vs Regression
Conclusion
With the above discussion, it is evident, that there is a big difference between these two mathematical concepts, although these two are studied together. Correlation is used when the researcher wants to know that whether the variables under study are correlated or not, if yes then what is the strength of their association. Pearson’s correlation coefficient is regarded as the best measure of correlation. In regression analysis, a functional relationship between two variables is established so as to make future projections on events.
kelil says
Liked
Feleke Assefa says
It is worth appreciating ! The way of expressing is very clear and to the point ! I thank you !
Vijaykumar Mali says
Nice Explanation, It’s very clear..
Thanks so much…..
Misbah Zaffar says
Thanks for the article… It’s truly helpful… 🥀
Valence says
It clears up my confusion. Thanks!
Surbhi S says
Thank you all the readers for appreciating the article. 🙂
MakKnight says
Kindly elaborate how price and demand are negatively correlated. I thought a increase in demand triggers an increase in price…
Surbhi S says
An increase in price leads to the decrease in the demand for commodity, and that is why, they are negatively correlated.
Tim John Joseph says
Great explanation, especially the comparison table. Was able to understand the differences very clearly. Thankyou 🙂
khushboo says
This was fabulous. My doubt is completely clear.
Sandeep says
Well elaborated..thanx
OsamaSomy says
done…!
Good jod.
thanks..
Hadi says
Thanks for the clear explanation.
PARTHA says
IT CLEARS MY CONFUSION
Suprabha Thapaliya says
Very useful
Dhruba Timalsina says
really appreciated
Rose says
It is really great and helpful explanation thanks👍
Chhavi Prakash Mahto says
Very useful and knowledgeable article.
Surbhi S says
Thanks for appreciating, your views mean a lot to us, keep visiting. 🙂
junaid ramzan says
worth appreciating… love you
Badang says
It makes it much simpler to distinguish between correlation and regression. Thanks
Akash Maity says
This is too easy to understand
Rio Hemara says
Thank you, It is very useful. very simple to understand.
Muthama Matsitsi says
Well hammered.
Its now crystal clear to me.
Have been confusing the two throughout
Neeraj Dewangan says
Hi Surbhi,
Thanks for explaining the difference thoroughly with proper points. In statistics, it’s very important to understand each and every concept clearly so as to work properly. I am studying R and faced the difficulty to understand. I am glad that ur this piece of write up helped me to understand the concept.
Thanks again!!
You should write more often in statistics also, and keep up the good work.
Mostafa says
Is it possible to use correlation for binary data?
ashok gaudel says
The way it is expressed is awesome , I thank you
Auwal Ahmed says
This is amazing. Thank you so much!
Otu Unor says
Succinctly explained. Nice one!
Ngozi Ogbuju says
very very insightful and well explanatory
Gianfranco says
Thank you, quite clear!
Richard Sithole says
Short and to the point
R.Rambabu says
Hi, your sharing information was excellent 👌 and i,m visiting your site regularly why because you are giving amazing information and straight forward information, Thankyou.
Ana Carol says
Great explanation on the difference between correlation and regression!
AZMATULLAH KHAN says
good work