Difference Between Variance and Standard Deviation

Dispersion indicates the extent to which observations deviate from an appropriate measure of central tendency. Measures of dispersion fall into two categories i.e. an absolute measure of dispersion and relative measure of dispersion. Variance and standard deviation are two types of an absolute measure of variability; that describes how the observations are spread out around the mean. Variance is nothing but the average of the squares of the deviations,

Unlike, standard deviation is the square root of the numerical value obtained while calculating variance. Many people contrast these two mathematical concepts. So, this article makes an attempt to shed light on the important difference between variance and standard deviation.

Content: Variance Vs Standard Deviation

1. Comparison Chart
2. Definition
3. Key Differences
4. Illustration
5. Similarities
6. Conclusion

Comparison Chart

Basis for Comparison	Variance	Standard Deviation
Meaning	Variance is a numerical value that describes the variability of observations from its arithmetic mean.	Standard deviation is a measure of dispersion of observations within a data set.
What is it?	It is the average of squared deviations.	It is the root mean square deviation.
Labelled as	Sigma-squared (σ^2)	Sigma (σ)
Expressed in	Squared units	Same units as the values in the set of data.
Indicates	How far individuals in a group are spread out.	How much observations of a data set differs from its mean.

Definition of Variance

In statistics, variance is defined as the measure of variability that represents how far members of a group are spread out. It finds out the average degree to which each observation varies from the mean. When the variance of a data set is small, it shows the closeness of the data points to the mean whereas a greater value of variance represents that the observations are very dispersed around the arithmetic mean and from each other.
For unclassified data: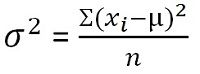

For grouped frequency distribution: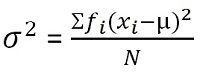

Definition of Standard Deviation

Standard deviation is a measure that quantifies the amount of dispersion of the observations in a dataset. The low standard deviation is an indicator of the closeness of the scores to the arithmetic mean and a high standard deviation represents; the scores are dispersed over a higher range of values.
For unclassified data: 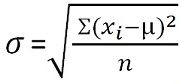For grouped frequency distribution: 

Illustration

Marks scored by a student in five subjects are 60, 75, 46, 58 and 80 respectively. You have to find out the standard deviation and variance.
First of all, you have to find out the mean,

So the average (mean) marks are 63.8
Now calculate the variance

X	A	(x-A)	(X-A)^2
60	63.8	-3.8	14.44
75	63.8	11.2	125.44
46	63.8	-17.8	316.84
58	63.8	5.8	33.64
80	63.8	16.2	262.44

Where, X = Observations
A = Arithmetic Mean

So the variance is 150.56

And Standard deviation is –

Similarities

• Both variance and standard deviation are always positive.
• If all the observations in a data set are identical, then the standard deviation and variance will be zero.

Conclusion

These two are basic statistical terms, which are playing a vital role in different sectors. Standard deviation is preferred over mean as it is expressed in the same units as those of the measurements while the variance is expressed in the units larger than the given data set.